“Numbers are the highest degree of knowledge. It is knowledge itself.” – Plato
We live in a mathematical world. Whenever we decide on a purchase, choose an insurance or health plan, or use a spreadsheet, we rely on mathematical understanding.
The level of mathematical skills needed in the workplace has increased dramatically. In such a world, those who understand and can do mathematics will have opportunities that others do not. Mathematical competence opens doors to productive futures.
Students have different abilities, needs, and interests. Yet everyone needs to be able to use mathematics in his or her personal life, in the workplace, and in further study. All students deserve an opportunity to understand the power and beauty of mathematics. Students need to learn a new set of mathematics basics that enable them to compute fluently and solve problems creatively and resourcefully.
The mission of the Mathematics Department is to develop in our students the mathematical abilities they will need to become productive citizens. We are committed to providing curriculum, instruction, and assessment that will actively involve students in constructing and applying mathematical ideas, to solve problems and enable students to express the mathematical connections in the world around us with algebraic, geometric, or numeric representations.
In Math, there are no problems, only solutions.
**Students are required to complete 3 credits of math to graduate. District 230 works closely with partner schools to ensure each student has a smooth and successful math transition.
Student Calculator Needs
A Graphing Calculator is required for most math courses. The required graphing calculator is the TI-84+.
Additional Math Support and Extended Courses
Division chairs, teachers, and counselors work with students to determine additional support needed in order to develop certain skills or provide additional support to ensure students are successful in the curriculum. Extended course opportunities are prescribed to students when they have been identified as needing additional support to master the learning targets of the academic course. If a student fails a course, summer school courses may be necessary in order for students to stay on track for graduation in four years.
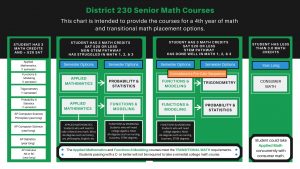
Senior Year Math Courses
(click on the image to enlarge)
AP Calculus AB
Prerequisite: Honors Math 3 with a grade of C or higher and teacher recommendation
Grade Level: 11-12
Semesters: 2
Credits: 1
Students will study differential and integral calculus. This course follows the College Board’s Advanced Placement curriculum and as such is extremely rigorous and fast paced. Students may receive college credit in Calculus by qualifying on an examination administered by the College Entrance Examination Board. Students in this course may be required to complete a summer assignment to assist in the retention of previously learned material.
AP Calculus BC
Prerequisite: Honors Math 3 with a grade of B or higher and teacher recommendation or successful completion of AP Calculus AB.
Grade Level: 11-12
Semesters: 2
Credits: 1
Students will study the equivalent of Calculus I and II as would be offered at any college or university. The course will cover both differential and integral calculus with further applications to vectors, polar functions, and series. This course follows the College Board’s Advanced Placement rigorous curriculum framework. Students may receive college credit for up to two semesters of Calculus by earning scores set by the receiving universities/colleges on the AP examination administered by the College Board Examination Board. Students should be aware the pace and content of the course exceed that of AP Calculus AB. Students in this course may be required to complete a summer assignment to assist in the retention of previously learned material.
AP Computer Science JAVA
Prerequisite: Completion of Honors Math 2 or Math 3 with a B or better or concurrent registration in Honors Math 3
Grade Level: 10-12
Semesters: 2
Credits: 1
Students will study the structured program and the syntax of computer languages. Object-oriented programming techniques will be studied and implemented in all programs. This course follows the College Board’s Advanced Placement curriculum and as such is extremely rigorous and fast-paced. Students may receive college credit in Computer Science by qualifying on an examination administered by the College Entrance Examination Board.
Please note that while this course is located in our math department some colleges don’t view this as a math course. Please consult with any college where you may be applying to see if this course meets their math requirement.
AP Computer Science Principles
Grade: 9-12
Semester: 2
Prerequisite: None
The AP Computer Science Principles course is designed to be equivalent to a first-semester introductory college computing course. In this course, students will develop computational thinking skills vital for success across all disciplines, such as using computational tools to analyze and study data and working with large data sets to analyze, visualize, and draw conclusions from trends. The course is unique in its focus on fostering student creativity. Students are encouraged to apply creative processes when developing computational artifacts and to think creatively while using computer software and other technology to explore questions that interest them. They will also develop effective communication and collaboration skills, working individually and collaboratively to solve problems and discussing and writing about the importance of these problems and their impacts on their community, society, and the world.
Please note that while this course is located in our math department some colleges don’t view this as a math course. Please consult with any college where you may be applying to see if this course meets their math requirement.
AP Statistics
Prerequisite: Completion – Completion of Honors Math 2 or Math 3 with a B or better or concurrent registration in Honors Math 3
Grade Level: 10-12
Semesters: 2
Credits: 1
Students will be introduced to the major concepts and tools for collecting, analyzing, and drawing conclusions from data. The four major themes presented in the course are exploring data, planning a study, anticipating patterns, and statistical inference. There is a large written language component to this class. This course follows the College Board’s Advanced Placement curriculum and, as such, is rigorous and fast-paced. Students may receive college credit in Statistics. Students in this course may be required to complete a summer assignment to assist in the retention of previously learned material.
Please note that while this course is located in our math department, some colleges don’t view this as a math course. Please consult with any college where you may be applying to see if this course meets their math requirement.
Applied Mathematics
Prerequisite: This course is for students who have successfully completed three years of math to meet the graduation requirements. Students who earn a grade of C- or better will obtain a portability code on their transcript. Grade Level: 12
Semesters: 1
Credits: .5
Students will be engaged in authentic, application-based math problems that will forge connections between classroom content and students’ lives. Topics include managing personal finances, financial decision-making in a variety of scenarios, and using math for post-secondary decisions. The course will end with a capstone project where students will display their understanding of how to apply mathematics to their daily lives after high school.
This course meets the state requirement to allow entry into a 100 college-level math class, without a STEM focus. A student needs to earn a C- or higher to meet this state requirement.
Calculus 3 & Linear Algebra
Prerequisite: An earned 3, 4, or 5 on AP Calculus BC Exam or a B or higher in a college or university credit in Calculus I and Calculus II
Grade Level: 11-12
Semesters: 2
Credits: 1
This yearlong course is split into two separate college-level courses. The fall semester course of study is on Multivariable Calculus. Topics of study include vector algebra, curves, and surfaces in space, functions of several variables, partial derivatives, the chain rule, the gradient vector, Lagrange multipliers, multiple integrals, volume, surface area, the Change of Variables Theorem, line integrals, surface integrals, Green’s Theorem, the Divergence Theorem, and Stokes’ Theorem. The spring semester course of study is on Linear Algebra. Topics of study include Gaussian elimination, matrix algebra, linear independence, span, basis, linear transformations, determinants, eigenvalues, eigenvectors, and diagonalization. Dual credit is available for a separate course fee with the college/university.
Consumer Math
Prerequisite: This course is only for students that have not successfully passed three years of math
Grade Level: 12
Semesters: 2
Credits: 1
Students will learn concepts and problem-solving techniques necessary to successfully deal with consumer and career applications. Students will work on authentic, application-based math problems to make connections between classroom content and students’ lives. Topics include managing personal finances and understanding financial decision-making in a variety of scenarios by using math to make informed post-secondary decisions.
Students may take one semester if needed to complete the three-year graduation requirement with the successful completion of 2.5 years of math. After the first semester’s successful completion of Consumer Math, they could take an additional math transitional course in an attempt to gain the math portability code. Applied Mathematics would be the suggested second-semester course.
Functions and Modeling
Prerequisite: This course is for students who have successfully completed three years of math to meet the graduation requirements. Students who earn a grade of C- or better will obtain a portability code on their transcript.
Grade: 12
Semester: 1
Credits: .5
This STEM-driven, function-based approach to introductory college algebra includes linear, polynomial, rational, radical, and exponential functions. Many phenomena in the world around us can be modeled with functions, so this course takes a more deliberate approach to these understandings. While this course is designed for students who intend to enter a career field of science, technology, engineering, and/or mathematics, it will also benefit students that will use similar skills (additional related careers can be found in health care, information technology, business management, and administration, etc.).
This course meets the state requirement to allow entry into a 100 college-level math class, without a STEM focus. A student needs to earn a C- or higher to meet this state requirement.
Honors Math 1
Prerequisite: Department Chair Recommendation
Grade Level: 9
Semesters: 2
Credit: 1
Topics include recognizing and developing patterns using tables, graphs, and equations. Mathematical modeling is stressed as a methodology for approaching the solution to problems. Students will explore operations on algebraic expressions and apply mathematical properties to algebraic equations. Students will problem-solve using equations, graphs, and tables and investigate linear and exponential relationships, including comparing and contrasting options and decision-making using algebraic models. Topics from two-dimensional Geometry are integrated into this curriculum. This includes congruence, construction, and proof, as well as applying geometry to the coordinate plane. Instruction in the area of data analysis is introduced. Technology will be used for guided practice. Math 1 Honors also includes standards from Pre-Calculus courses so that when a student completes the Honors Math sequence they will be prepared for AP Calculus.
Honors Math 2
Prerequisite: Honors Math 1 or teacher recommendation
Grade Level: 10
Semesters: 2
Credit: 1
Honors Math 2 topics include quadratic expressions, equations, and functions; comparing their characteristics and behavior to those of linear and exponential relationships from Math I. This course includes standards from the conceptual categories of Number and Quantity, Algebra, Functions, Geometry, and Statistics and Probability. The scope of Honors Math 2 is limited to quadratic expressions and functions, and some work with absolute values, steps, and functions that are piecewise defined.
In Honors Math 2, instructional time will focus on seven critical areas: (1) extending the laws of exponents to rational exponents; (2) comparing key characteristics of quadratic functions with those of linear and exponential functions; (3) creating and solving equations and inequalities involving linear, exponential, and quadratic expressions; (4) extending work with probability; (5) establishing criteria for similarity of triangles based on dilations and proportional reasoning, (6) Trigonometric identities, and (7) circles. Honors Math 1 also includes standards from Pre-Calculus courses so that when a student completes the Honors Math sequence they will be prepared for AP Calculus.
Honors Math 3
Prerequisite: Successful Completion of Honors Math 2 or teacher recommendation
Grade Level: 11, 12
Semesters: 2
Credits: 1
Honors Math 3 will integrate and apply the mathematics they have learned from their earlier courses. This course includes standards from the conceptual categories of Number and Quantity, Algebra, Functions(Rational, Logarithmic, Trigonometric, and Polynomial), and Geometry. Students synthesize and generalize what they have learned about a variety of function families. They extend their work with exponential functions to include solving exponential equations with logarithms. They explore the effects of transformations on graphs of diverse functions, including functions arising in an application, in order to abstract the general principle that transformations on a graph always have the same effect regardless of the type of underlying function. They identify appropriate types of functions to model a situation, adjust parameters to improve the model, and they compare models by analyzing the appropriateness of fit and making judgments about the domain over which a model is a good fit. The description of modeling as “the process of choosing and using mathematics and statistics to analyze empirical situations, to understand them better, and to make decisions” is at the heart of Honors Math 3.
In Honors Math 3 instructional time should focus on three critical areas:(1) expand understanding of functions to include polynomial, rational, and radical functions;(2) expand right triangle trigonometry to include general triangles; and(3) consolidate functions and geometry to create models and solve contextual problems.
Math 1
Prerequisite: None
Grade Level: 9
Semesters: 2
Credit: 1
Topics include recognizing and developing patterns using tables, graphs, and equations. Mathematical modeling is stressed as a methodology for approaching the solution to problems. Students will explore operations on algebraic expressions and apply mathematical properties to algebraic equations. Students will problem-solve using equations, graphs, and tables and investigate linear and exponential relationships, including comparing and contrasting options and decision-making using algebraic models. Topics from two-dimensional Geometry are also integrated into this course including congruence, construction, and proof, as well as applying geometry to the coordinate plane. Instruction in the area of data analysis is introduced. Technology will be used for guided practice.
Math 2
Prerequisites: Math 1
Grade Level: 10
Semesters: 2
Credit: 1
Math 2 topics include quadratic expressions, equations, and functions; comparing their characteristics and behavior to those of linear and exponential relationships from Math I. This course includes standards from the conceptual categories of Number and Quantity, Algebra, Functions, Geometry, and Statistics and Probability. The scope of Math 2 is limited to quadratic expressions and functions, and some work with absolute values, steps, and functions that are piecewise-defined.
In Math 2, instructional time will focus on seven critical areas: (1) extending the laws of exponents to rational exponents; (2) comparing key characteristics of quadratic functions with those of linear and exponential functions; (3) creating and solving equations and inequalities involving linear, exponential, and quadratic expressions; (4) extending work with probability; (5) establishing criteria for similarity of triangles based on dilations and proportional reasoning, (6) Trigonometric identities, and (7) circles.
Math 3
Prerequisite: Math 2
Grade Level 11-12
Semester: 2
Credit: 1
Math 3 will integrate and apply the mathematics they have learned from their earlier courses. This course includes standards from the conceptual categories of Number and Quantity, Algebra, Functions(Rational, Logarithmic, Trigonometric, and Polynomial), and Geometry. Students synthesize and generalize what they have learned about a variety of function families. They extend their work with exponential functions to include solving exponential equations with logarithms. They explore the effects of transformations on graphs of diverse functions, including functions arising in an application, in order to abstract the general principle that transformations on a graph always have the same effect regardless of the type of underlying function. They identify appropriate types of functions to model a situation, adjust parameters to improve the model, and they compare models by analyzing the appropriateness of fit and making judgments about the domain over which a model is a good fit. The description of modeling as “the process of choosing and using mathematics and statistics to analyze empirical situations, to understand them better, and to make decisions” is at the heart of this Mathematics III course.
In Math 3 instructional time should focus on three critical areas:(1) expand understanding of functions to include polynomial, rational, and radical functions;(2) expand right triangle trigonometry to include general triangles; and(3) consolidate functions and geometry to create models and solve contextual problems
Math in Construction
Prerequisite: Math 2
Grade Level: 12
Semesters: 2
Credits: 2
Math in Construction is an interdisciplinary course that integrates Mathematics and Construction
topics through the building of a significant construction project. Math in Construction is taught
by both Math and Technology & Engineering Education (TEE) teachers. The mathematics
content matches the Math 1-3 curriculum taught in the Mathematics Department. Students will
gain hands-on experience in construction with additional emphasis on teamwork,
problem-solving, and the promotion of STEM education. This course will be taught in a
two-period block, students earn credit for Math and House Wiring/Building Construction (CTE).
Students must enroll in Math in Construction to fulfill the block. Students are required to enroll in
both semesters. Math topics covered during Math in Construction will include, but are not limited
to: a review of arithmetic and fractions, order of operations, rounding, factoring, solving
equations, surface area, volume, ratios, proportions, percents, scale factors, slope, graphing
and writing equations of lines, angle properties, triangle properties, polygon properties,
congruent and similar figure properties, and circle properties. Throughout the year, students will
work on construction projects that embed these math skills.
Probability and Statistics
Prerequisite: This course is for students who have successfully completed three years of math and meet the requirements to take a 100-level college math class.
Grade Level: 12
Semesters: 1
Credits: .5
Students will be introduced to modern statistics and probability theory and the basic statistical ideas needed in such areas as sociology, business, economics, ecology, education, medicine, psychology, and mathematics. This course includes study in both descriptive and inferential statistics.
Trigonometry
Prerequisite: This course is for students who have successfully completed three years of math and meet the requirements to take a 100-level college math class.
Grade Level: 12
Semesters: 1
Credits: .5
Students will explore right triangle trigonometry, as well as trigonometric graphs and various trigonometric identities. This course is more geared toward students pursuing a career in a STEM-based field where they may find the use of more rigorous mathematics. This semester course, combined with a semester course in Functions and Modeling, constitutes an academic Pre-Calculus course.